[SS] Differential Equation : RL System
SS 2022. 9. 14. 17:00다음의 RL회로를 미분방정식으로 풀기
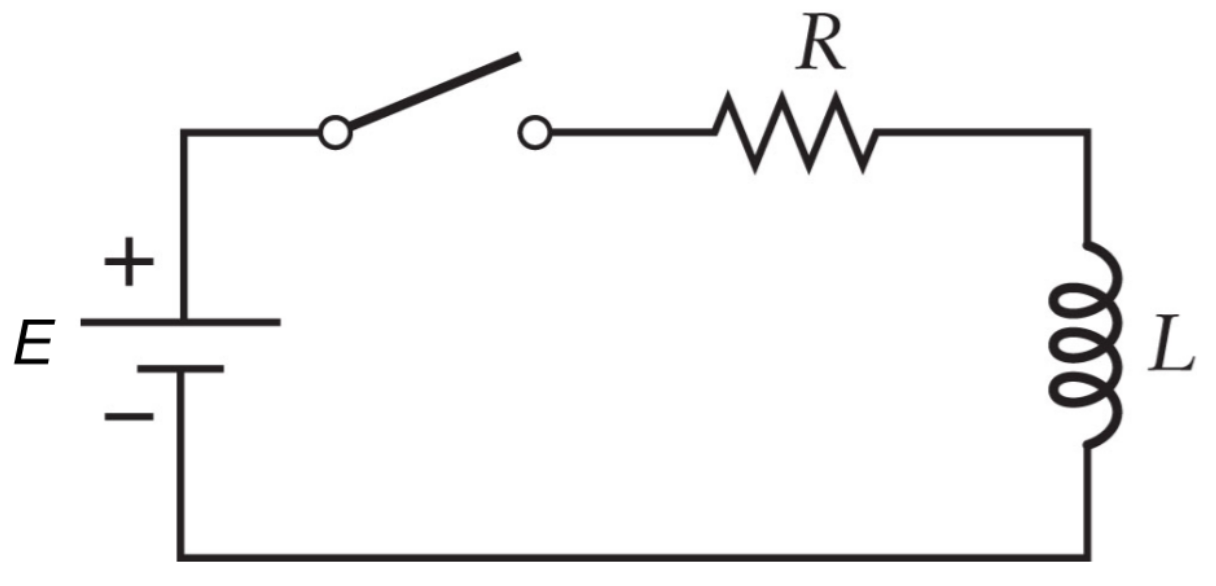
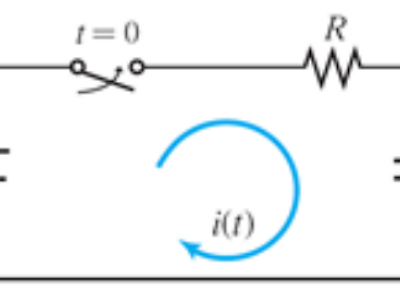
$t=0$에서 스위치가 닫힘.
위의 회로에서 입출력 및 초기조건은 다음과 같음.
- input : $E$ (voltage)
- output : $i(t)$ (current)
- initial condition : $i(t=0)=i_0=0$ (inductor L의 $t=0$에서의 전류.)
1. Differential Equation
KVL에 의하여 다음이 성립
$$\begin{aligned}
L\frac{di(t)}{dt}+Ri(t) &= E \\
\frac{di(t)}{dt}+\frac{R}{L}i(t) &= \frac{E}{L}
\end{aligned}$$
2. Homogeneous solution
$$\begin{aligned}
\frac{di_h(t)}{dt}+\frac{R}{L}i_h(t) &= 0 \\
\frac{di_h(t)}{dt} &= -\frac{R}{L}i_h(t) \\
\frac{1}{i_h(t)}di_h(t) &= -\frac{R}{L}dt \\
\displaystyle{\int_{A}^{i_h(t)} \frac{1}{i_h(\tau)}di(\tau)} &= \displaystyle{-\frac{R}{L} \int_0^{t} d\tau} \\
\left[ \ln i_h(\tau) \right] _{A}^{i_h(t)} &= \left[ -\frac{R}{L} \tau \right]_0^t \\
\ln i_h(t) - \ln A &= -\frac{R}{L} t \\
\ln \frac{i_h(t)}{A} &= -\frac{R}{L} t \\
\frac{i_h(t)}{A} &= e^{-\frac{R}{L} t} \\
i_h(t) &= A e^{-\frac{R}{L} t} \\
\end{aligned}$$
위와 같이 푸는 방법을 separtion of variable 이라고 부름!
특성방정식으로 풀면 다음과 같음.
$$\begin{aligned} \frac{di_h(t)}{dt}+\frac{R}{L}i_h(t) &= 0 \\ Di_h(t)+\frac{R}{L}i_h(t) &= 0 \\ (D+\frac{R}{L})i_h(t) &= 0 \\ \lambda &= -\frac{R}{L} \\ i_h(t) &= A e^{-\frac{R}{L} t} \\ \end{aligned}$$
3. Particular solution
$$\frac{di(t)}{dt}+\frac{R}{L}i(t)= \frac{E}{L}$$
$\frac{E}{R}$은 상수이므로, particular solution $i_p(t)$도 상수의 형태를 가짐 (particular solution은 입력함수에 의해 그 형태가 결정됨을 기억할 것). 즉, 다음과 같은 식으로 표현 가능.
$$i_p(t)=n$$
이를 미분방정식에 대입하여 풀면, 다음과 같음.
$$\begin{aligned}
\frac{di(t)}{dt}+\frac{R}{L}i(t) &= \frac{E}{L} \\
\frac{dn}{dt}+\frac{R}{L}n &= \frac{E}{L} \\
0+\frac{R}{L}n &= \frac{E}{L} \\
n &= \frac{E}{R} \\
\therefore i_p(t) &= \frac{E}{R}
\end{aligned}$$
4. Complete solution
complete soluton $i(t)$는 다음과 같이 homogeneous solution과 particular solution의 합임.
$$\begin{aligned}
i(t) &= i_h(t) + i_p(t) \\
&=A e^{-\frac{R}{L} t} + \frac{E}{R}
\end{aligned}$$
위의 solution의 식에서 우리가 모르는 상수는 $A$임. ($R$,$L$,$E$는 회로에서 주어진 상수)
$A$를 구하기 위해, 초기조건을 이용한다.
$$\begin{aligned}
i_0=i(0)&=A e^{-\frac{R}{L} 0} + \frac{E}{R}=0 \\
\therefore A&=-\frac{E}{R}
\end{aligned}$$
즉, 출력 $i(t)$는 다음과 같음.
$$\begin{aligned}
i(t) &=-\frac{E}{R} e^{-\frac{R}{L} t} + \frac{E}{R} \\
&=\frac{E}{R} (1-e^{-\frac{R}{L} t})
\end{aligned}$$
'SS' 카테고리의 다른 글
| [SS] Differential Equation : RC System (0) | 2022.09.14 |
|---|---|
| [SS] Example : Sampling function and Sinc function. (2) | 2022.09.05 |
| [SS] Example : Sampling function and Sinc function. (0) | 2022.09.02 |